Learning PDEs for Image Restoration via Optimal Control

Abstract

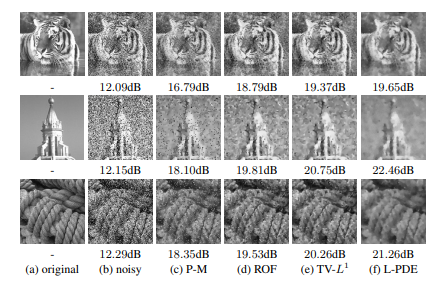
Partial differential equations (PDEs) have been successfully applied to many computer vision and image processing problems. However, designing PDEs requires high mathematical skills and good insight into the problems. In this paper, we show that the design of PDEs could be made easier by borrowing the learning strategy from machine learning. In our learning-based PDE (L-PDE) framework for image restoration, there are two terms in our PDE model: (i) a regularizer which encodes the prior knowledge of the image model and (ii) a linear combination of differential invariants, which is data-driven and can effectively adapt to different problems and complex conditions. The L-PDE is learnt from some input/output pairs of training samples via an optimal control technique. The effectiveness of our L-PDE framework for image restoration is demonstrated with two exemplary applications: image denoising and inpainting, where the PDEs are obtained easily and the produced results are comparable to or better than those of traditional PDEs, which were elaborately designed.